Question

A company makes auto batteries. They claim that 84 % of their LL70 batteries are good for 70 months or longer. Assume that this claim is true. Let p^ be the proportion in a random sample of 60 such batteries that are good for 70 months or more.

(a) What is the probability that this sample proportion is within 0.03 of the population proportion? Round your answer to two decimal places.
(b) What is the probability that this sample proportion is less than the population proportion by 0.05 or more? Round your answer to two decimal places.

Answer 1

(a) The probability that this sample proportion is within 0.03 of the population proportion is 0.48.

(b) The probability that this sample proportion is less than the population proportion by 0.05 or more is 0.86.

Explanation:

According to the Central limit theorem, if from an unknown population large samples of sizes n > 30, are selected and the sample proportion for each sample is computed then the sampling distribution of sample proportion follows a Normal distribution.

The mean of this sampling distribution of sample proportion is:

`\mu_(\hat p)=p`

The standard deviation of this sampling distribution of sample proportion is:

`\sigma_(\hat p)=\sqrt{(p(1-p))/(n)}`

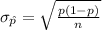
The information provided is:

n = 60

p = 0.84

Since the sample size is quite large, the Central limit theorem can be used to approximate the sampling distribution of sample proportion.

The mean and standard deviation are:

`\mu_(\hat p)=p=0.84\\\\\sigma_(\hat p)=\sqrt{(p(1-p))/(n)}=\sqrt{(0.84(1-0.84))/(60)}=0.047`

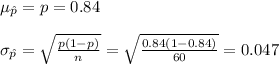
(a)

Compute the probability that this sample proportion is within 0.03 of the population proportion as follows:

`P(-0.03<\hat p-p<0.03)=P((-0.03)/(0.047) <\hat p-p<(0.03)/(0.047))`

`=P(-0.64<Z<0.64)\\=0.4778\\\approx0.48`

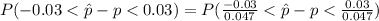
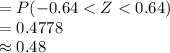
*Use a z-table.

Thus, the probability that this sample proportion is within 0.03 of the population proportion is 0.48.

(b)

Compute the probability that this sample proportion is less than the population proportion by 0.05 or more as follows:

`P(\hat p-p\geq -0.05)=P((\hat p-p)/(\sigma_(\hat p))>(-0.05)/(0.047))`

`=P(Z>-1.06)\\=P(Z<1.06)\\=0.8554\\\approx0.86`

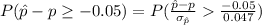
*Use a z-table.

Thus, the probability that this sample proportion is less than the population proportion by 0.05 or more is 0.86.