Question

You are given the polar curve r=eθ.(a) List all of the points (r,θ) where the tangent line is horizontal. In entering your answer, list the points starting with the smallest value of r and limit yourself to 1≤r≤1000 ( note the restriction on r!) and 0≤θ<2π. If two or more points share the same value of r, list those starting with the smallest value of θ. If any blanks are unused, type an upper-case "N" in them.

Answer 1

`(e^{(3\pi)/(4)},(3\pi)/(4)), (e^{(7\pi)/(4)},(7\pi)/(4))`.

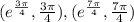Explanation:

REcall that given a parametric curve x(t),y(t), the tangent's line slope is given by

`(dy)/(dx) = ((dy)/(dt))/((dx)/(dt))`

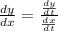
To find where the tangent line is horizontal, we must find where the slope is 0. That is, to find the values of t for which
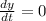`(dy)/(dt) =0` and
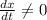`(dx)/(dt)\\eq0` at the same time.

Consider the polar curve
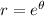`r=e^(\theta)`. If we use the polar coordinates, we have that
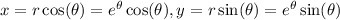`x = r\cos(\theta) = e^(\theta)\cos(\theta), y = r\sin(\theta) = e^(\theta)\sin(\theta)`, which gives us a parametric curve with parameter
`\theta`. So, let us use the above to find the desired points.

We have that

`(dy)/(dt) = e^(\theta)(\sin(\theta)+\cos(\theta))`

`(dx)/(dt) = e^(\theta)(\cos(\theta)-\sin(\theta))`

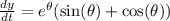
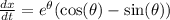
Recall that the function
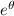`e^\theta` is never 0, so, for us to have the derivative of y to be 0, we must have that
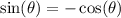`\sin(\theta) = -\cos(\theta)`. Note that if this happens, the derivative of x is different from 0. So, we must solve the following equation in the interval
`\theta \in [0,2\pi]`.

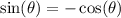`\sin(\theta) = -\cos(\theta)`, which is equivalent to
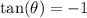`\tan(\theta) = -1`. Which gives us
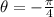`\theta=-(\pi)/(4)`. This solution is out of our desired interval, then , using the fact that tangent is a periodic function with period pi, we can find the solutions in the desired interval by adding multiples of pi. Thus, the desired solutions are

`\theta_1 = (-\pi)/(4)+\pi = (3\pi)/(4), \theta_2 = (-\pi)/(4)+2\pi = (7\pi)/(4)`

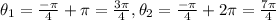
Note that
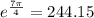`e^{(7\pi)/(4)} = 244.15` and
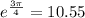`e^{(3\pi)/(4)} = 10.55`,so both solutions are inside the restriction.