Question

n 19451945​, an organization asked 14031403 randomly sampled American​ citizens, "Do you think we can develop a way to protect ourselves from atomic bombs in case others tried to use them against​ us?" with 737737 responding yes. Did a majority of the citizens feel the country could develop a way to protect itself from atomic bombs in 19451945​? Use the alpha equals 0.01α=0.01 level of significance.

Answer 1

We conclude that majority of the citizens does not feel the country could develop a way to protect itself from atomic bombs.

Explanation:

We are given that an organization asked 1403 randomly sampled American​ citizens, "Do you think we can develop a way to protect ourselves from atomic bombs in case others tried to use them against​ us?" with 737 responding yes.

We have to conduct a test to determine that a majority of the citizens feel the country could develop a way to protect itself from atomic bombs in 1945.

Let p = percentage of citizens feel the country could develop a way to protect itself from atomic bombs in 1945.

SO, Null Hypothesis,
`H_0` : p
`\leq` 50% {means that majority of the citizens does not feel the country could develop a way to protect itself from atomic bombs}

Alternate Hypothesis,
`H_A` : p > 50% {means that majority of the citizens feel the country could develop a way to protect itself from atomic bombs}

The test statistics that will be used here is One-sample z proportion statistics;

T.S. =
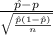`\frac{\hat p-p}{{\sqrt{(\hat p(1-\hat p))/(n) } } } }` ~ N(0,1)

where,
`\hat p` = proportion of citizens responding yes in a sample of 1403 citizens =
`(737)/(1403)`

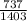
n = sample of citizens = 1403

So, test statistics =
`\frac{(737)/(1403) -0.50}{{\sqrt{((737)/(1403)(1-(737)/(1403)))/(1403) } } } }`

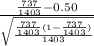
= 1.898

The value of the test statistics is 1.898.

Now at 1% significance level, the z table gives critical value of 2.3263 for right-tailed test. Since our test statistics is less than the critical value of z as 1.898 < 2.3263, so we have insufficient evidence to reject our null hypothesis as it will not fall in the rejection region due to which we fail to reject our null hypothesis.

Therefore, we conclude that majority of the citizens does not feel the country could develop a way to protect itself from atomic bombs.