Answer:
For this case we want to find this probability:
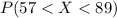
And we can calculate the number of deviations from the mean for the limits using the z score formula given by:

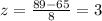
We know that within one deviation from the mean we have 68% of the values and on each tail we have (100-68)/2 % = 16%. And within 3 deviations we have 99.7% of the values and on each tail we have (100-99.7)/2% = 0.15%.
And the percentage desired would be:
(100%-0.15%) - 16% = 83.85%
Explanation:
Previous concepts
The empirical rule, also known as three-sigma rule or 68-95-99.7 rule, "is a statistical rule which states that for a normal distribution, almost all data falls within three standard deviations (denoted by σ) of the mean (denoted by µ)".
Let X the random variable who representt the number of potholes
From the problem we have the mean and the standard deviation for the random variable X.
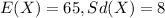
So we can assume

On this case in order to check if the random variable X follows a normal distribution we can use the empirical rule that states the following:
• The probability of obtain values within one deviation from the mean is 0.68
• The probability of obtain values within two deviation's from the mean is 0.95
• The probability of obtain values within three deviation's from the mean is 0.997
Solution to the problem
For this case we want to find this probability:
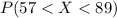
And we can calculate the number of deviations from the mean for the limits using the z score formula given by:

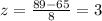
We know that within one deviation from the mean we have 68% of the values and on each tail we have (100-68)/2 % = 16%. And within 3 deviations we have 99.7% of the values and on each tail we have (100-99.7)/2% = 0.15%.
And the percentage desired would be:
(100%-0.15%) - 16% = 83.85%