Answer:


Since the p value is lower than the significance level
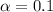 we have enough evidence to reject the null hypothesis and the claim for the manager makes sense.
we have enough evidence to reject the null hypothesis and the claim for the manager makes sense.
For the Ti84 preocedure we need to do this:
STAT> TESTS> 1-Z prop Test
And then we need to input the following values:
po= 0.58
x = 443 , n= 820
prop <po
And then calculate and we will get the same results
Explanation:
Data given and notation
n=820 represent the random sample taken
X=443 represent the people that had cell phones
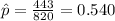 estimated proportion of people that had cell phones
estimated proportion of people that had cell phones
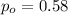 is the value that we want to test
is the value that we want to test
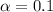 represent the significance level
represent the significance level
Confidence=90% or 0.90
z would represent the statistic (variable of interest)
 represent the p value (variable of interest)
represent the p value (variable of interest)
System of hypothesis
We need to conduct a hypothesis in order to test the claim that the true proportion is less than 0.58.:
Null hypothesis:

Alternative hypothesis:
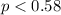
The statistic is given by:
 (1)
(1)
And replacing we got:
Since we have all the info requires we can replace in formula (1) like this:

Decision
The significance level provided
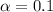 . Now we can calculate the p value
. Now we can calculate the p value
Since is a left tailed test the p value would be:

Since the p value is lower than the significance level
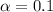 we have enough evidence to reject the null hypothesis and the claim for the manager makes sense.
we have enough evidence to reject the null hypothesis and the claim for the manager makes sense.
For the Ti84 preocedure we need to do this:
STAT> TESTS> 1-Z prop Test
And then we need to input the following values:
po= 0.58
x = 443 , n= 820
prop <po
And then calculate and we will get the same results