Question

The experimental apparatus shown in the figure above contains a pendulum consisting of a 0.66 kg ball attached to a string of length 0,60 m. The pendulum is released from rest at an angle of

60 degrees and collides with a ball of mass 0.22 kg initially at rest at the edge of a table. The 0.22 kg ball hits the floor a distance of 1.4 m from the edge of the table.
a. Calculate the speed of the 0.66 kg ball just before the collision
b. Calculate the speed of the 0.22 kg ball immediately after the collision
c. Calculate the speed of the 0.66 kg ball immediately after the collision
d. Indicate the direction of motion of the 0.66 kg ball immediately after the collision
To the left
to the right
e. Calculate the height to which the 0.66 kg ball rises after the collision
f. Based on your data, is the collision elastic?
Yes
No
Justify your answer.

Answer 1

To calculate the speed of the 0.66 kg ball just before the collision, we can use the conservation of mechanical energy.

Step-by-step explanation:

To calculate the speed of the 0.66 kg ball just before the collision, we can use the conservation of mechanical energy. The initial gravitational potential energy of the ball is equal to the final kinetic energy of the ball. Therefore, we have:

2 m g (1 - cos(theta)) = 0.5 m v^2

where m is the mass of the ball, g is the acceleration due to gravity, theta is the angle at which the ball is released, and v is the speed of the ball. Plugging in the values, we can solve for v to get the answer.

Answer 2

The problem is solved and the questions are answered below.

Step-by-step explanation:

a. To calculate the speed of the 0.66 kg ball just before the collision

V₀ + K₀ = V₁ + K₁

= mgh₀ = 1/2 mv₁²

where, h= r - r cosθ

V =
`√(2gh)`

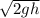
V = 2.42 m/s

b. Calculate the speed of the 0.22 kg ball immediately after the collision

y = y₀ + Vy₀t - 1/2 gt²

0 = 1.2 - 1/2 gt²

t = 0.495 s

x = x₀ + Vx₀t

1.4 = 0 + vx₀ (0.495)

Vx₀ = 2.83 m/s

C. To Calculate the speed of the 0.66 kg ball immediately after the collision

m₁ v₁ = m₁ v₃ + m₂ v₄

(0.66)(2.42) = (0.66) v₃ + (0.22)(2.83)

V₃ = 1.48 m/s

D. To Indicate the direction of motion of the 0.66 kg ball immediately after the collision is to the right.

E. To Calculate the height to which the 0.66 kg ball rises after the collision

V₀ + k₀ = V₁ + k₁

1/2 mv₀² = mgh₁

h₁ = v₀²/2 g

= 0.112 m

F. Based on your data, No the collision is not elastic.

Δk = 1/2 m₁v₃² =1/2 m₂v₄² - 1/2 m₁v₁²

= 1/2 (0.66)(1.48)² + 1/2 (0.22)(2.83)² - 1/2 (0.66)(2.42)²

= - 0.329 J

Hence, kinetic energy is not conserved.