Question

A marketing researcher wants to find a 96% confidence interval for the mean amount those visitors spend per person per day while visiting a theme park. She knows that the population standard deviation is $12. How large a sample should the researcher select so that the estimate will be within $4 of the population mean?

Answer 1

`n=((2.054(12))/(4))^2 =37.97 \approx 38`

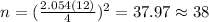
So then the minimum sample to ensure the condition given is n= 38

Explanation:

Notation

`\bar X` represent the sample mean for the sample

`\mu` population mean (variable of interest)

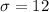`\sigma=12` represent the population standard deviation

n represent the sample size

ME = 4 the margin of error desired

Solution to the problem

When we create a confidence interval for the mean the margin of error is given by this formula:

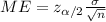`ME=z_(\alpha/2)(\sigma)/(√(n))` (a)

And on this case we have that ME =4 and we are interested in order to find the value of n, if we solve n from equation (a) we got:

`n=((z_(\alpha/2) \sigma)/(ME))^2` (b)

The critical value for 96% of confidence interval now can be founded using the normal distribution. The significance is
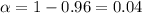`\alpha=1-0.96 =0.04`. And in excel we can use this formula to find it:"=-NORM.INV(0.02;0;1)", and we got
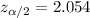`z_(\alpha/2)=2.054`, replacing into formula (b) we got:

`n=((2.054(12))/(4))^2 =37.97 \approx 38`

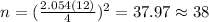
So then the minimum sample to ensure the condition given is n= 38