Question

2. Latoya opens a savings account and deposits $1,525. The interest rate is 2.73 percent. Interest is

compounded quarterly. How much interest will her deposit earn in one year?

Answer 1

To calculate the interest earned on the deposit, you can use the formula A = P(1+r/n)
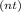`^{(nt)`, where A is the total amount after interest, P is the principal amount (initial deposit), r is the interest rate, n is the number of times interest is compounded per year, and t is the number of years.

Step-by-step explanation:

To calculate the interest earned on the deposit, we can use the formula A = P(1+r/n)
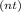`^{(nt)`, where A is the total amount after interest, P is the principal amount (initial deposit), r is the interest rate, n is the number of times interest is compounded per year, and t is the number of years.

In this case, Latoya's deposit is $1,525, the interest rate is 2.73%, and interest is compounded quarterly. We can plug these values into the formula:

A = 1525(1+0.0273/4)⁽⁴ˣ¹⁾

Using a calculator, we can evaluate this expression to find that the total amount after one year is approximately $1563.44.

The interest earned is therefore $1563.44 - $1525 = $38.44.

Answer 2

Step-by-step explanation:

Deposit = $1,525

Interest = 2.73%

Duration: 12 months

1,525/100 x 2.73/1

15.25 x 2.73

= 41.7 x 3

= $125.1