Question

A projectile with an initial velocity of 48 feet per second is launched from a building 190 feet tall. The path of the projectile is modeled using the equation h(t) = –16t2 + 48t + 190.

Answer 1

Answer: C

Step-by-step explanation: took the test

Answer 2

t = 5.25 seconds

Explanation:

Given that,

A projectile with an initial velocity of 48 feet per second is launched from a building 190 feet tall. The path of the projectile is modeled using the equation:

`h(t)=-16t^2+48t+190`

It is assumed to find the time when the projectile will hit the ground. When the projectile hit the ground, its height is equal to 0 such that,

`-16t^2+48t+190=0`

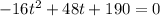
It forms a quadratic equation such that,

`t=\frac{-b+\sqrt{b^(2)-4ac } }{2a},\frac{-b-\sqrt{b^(2)-4ac } }{2a}\\\\t=\frac{-48+\sqrt{(48)^(2)-4* (-16)(190) } }{2* (-16)},\frac{-48-\sqrt{(48)^(2)-4* (-16)(190) } }{2* (-16)}\\\\t=-2.25, 5.25`

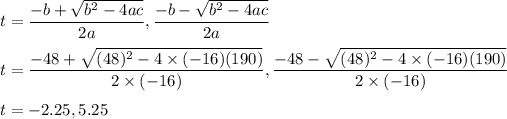
So, the projectile will hit the ground at t = 5.25 seconds.