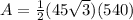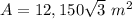Question

For each problem, use the formula for area of a polygon A = 12 ap , where a is the length of the

apothem and p is the perimeter of the polygon, to find the area of the polygon.
Show the exact values of a and p on your figure and give your answers as exact values - no rounding – no decimals. Show all your work clearly. All polygons represented are regular polygons.

Answer 1

Part 1) Triangle:
`P=24√(3)\ in` and
`A=48√(3)\ in^2`

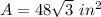
Part 2) Square:
`P=40√(2)\ ft` and
`A=200\ ft^2`

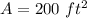
Part 3) Hexagon 1:
`P=90\ cm` and
`A=(675√(3))/(2))\ cm^2`

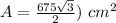
Part 4) Hexagon 2:
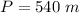`P=540\ m` and
`A=12,150√(3)\ m^2`

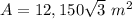
Explanation:

Part 1) we have an equilateral triangle

we know that

`A=(1)/(2)ap`

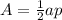
where

a is the apothem

P is the perimeter

step 1

Find the length side of the equilateral triangle

Let

b ----> the length side of triangle

we know that

The measure of each interior angle is 60 degrees

so

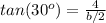`tan(30^o)=(4)/(b/2)` ---> by TOA (opposite side divided by the adjacent side)

`tan(30^o)=(1)/(√(3))`

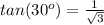
equate

`(4)/(b/2)=(1)/(√(3))`

`(8)/(b)=(1)/(√(3))`

`b=8√(3)\ in`

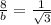
step 2

Find the perimeter

`P=3b`

`P=3(8√(3))=24√(3)\ in`

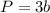
step 3

Find the area

`A=(1)/(2)(4)(24√(3))=48√(3)\ in^2`

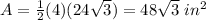
Part 2) we have a square

we know that

`A=(1)/(2)ap`

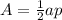
where

a is the apothem

P is the perimeter

step 1

Find the length side of the square

Let

b ----> the length side of the square

we know that

The diagonal is half the radius of the square

Applying the Pythagorean Theorem

`D^2=b^2+b^2`

`D^2=2b^2`

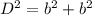
we have

`D=2a=2(10)=20\ ft`

`20^2=2b^2`

`b^2=200\\b=√(200)\ ft`

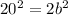
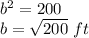
simplify

`b=10√(2)\ ft`

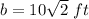
step 2

Find the perimeter

`P=4b=4(10√(2))=40√(2)\ ft`

step 3

Find the area

`A=(1)/(2)ap`

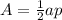
The apothem is half the length side of the square

`a=5√(2)\ ft`

`A=(1)/(2)(5√(2))(40√(2))`

`A=200\ ft^2`

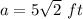
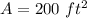
Part 3) we have a regular hexagon

we know that

`A=(1)/(2)ap`

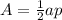
where

a is the apothem

P is the perimeter

step 1

Find the length side of the hexagon

we know that

The length side of a regular hexagon is equal to the radius

Let

b ----> the length side of the hexagon

we have

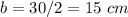`b=30/2=15\ cm` ----> the radius is half the diameter

step 2

Find the perimeter

`P=6b=6(15)=90\ cm`

step 3

Find the area

`A=(1)/(2)ap`

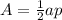
The apothem is the height of an equilateral triangle

Applying the Pythagorean Theorem

`b^2=(b/2)^2+a^2`

`15^2=(15/2)^2+a^2`

`a^2=225-(225/4)`

`a^2=675/4\\a=(√(675))/(2)\ cm`

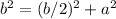
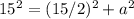
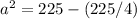
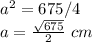
simplify

`a=(15√(3))/(2)\ cm`

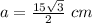
substitute in the formula of area

`A=(1)/(2)((15√(3))/(2))(90)`

`A=(675√(3))/(2))\ cm^2`

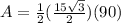
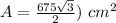
Part 4) we have a regular hexagon

we know that

`A=(1)/(2)ap`

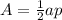
where

a is the apothem

P is the perimeter

step 1

Let

b ---> the length side of the hexagon

we have

`b=90\ m`

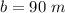
Find the perimeter

`P=6b=6(90)=540\ m`

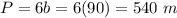
step 2

Find the area

`A=(1)/(2)ap`

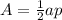
The apothem is the height of an equilateral triangle

Applying the Pythagorean Theorem

`b^2=(b/2)^2+a^2`

`90^2=(90/2)^2+a^2`

`a^2=8,100-2,025`

`a^2=6,075\\\\a=√(6,075)\ m`

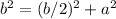
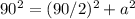
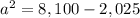
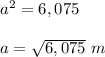
simplify

`a=45√(3)\ m`

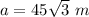
substitute in the formula of area

`A=(1)/(2)(45√(3))(540)`

`A=12,150√(3)\ m^2`