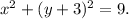Question

Please help!!!! I am about to fail !!

Answer 1

Given:

A circle with its center at (0, -3).

To find:

The standard equation for the given circle.

Solution:

The standard form of a circle is
`(x-h)^(2)+(y-k)^(2)=r^(2)`, where (h, k) is the center of the circle and r is the radius of the circle.

The center of the given circle is at (0, -3).

We need to determine the radius of the circle. The center is at (0, -3) and a point with the same y coordinate is (3, -3).

The radius of the circle
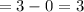`= 3-0=3` units.

So for the given circle, (h, k) is (0, -3) and r is 3 units.

So the equation becomes
`(x-0)^(2)+(y-(-3))^(2)=3^(2)`.

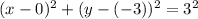The standard equation for the circle is
`x^(2)+(y+3)^(2)=9.`