Answer:
The distance the tornado traveled was approximately 205 miles.
Explanation:
The equation representing the relationship between the wind speed (in miles per hour) and the distance the tornado travels (in miles) is:
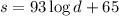
Compute the value of d for s = 280 as follows:
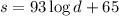
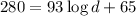
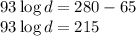

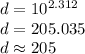
Thus, the distance the tornado traveled was approximately 205 miles.