Question

Find the area of the shaded sector. Round to the nearest tenth and use 3.142 or the pi button on your calculator for pi.

answers: (all answers are squared)

44.0 in2

66.0 in2

263.9 in2

22.0 in2

Answer 1

Given:

Given that the radius of the circle is 12 inches.

The angle of the shaded sector is 210°

We need to determine the area of the shaded sector.

Area of the shaded sector:

The area of the shaded sector can be determined using the formula,

`Area =((\theta)/(360)) \pi r^2`

Substituting
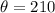`\theta=210` and r = 12, we get;

`Area =((210)/(360)) (3.142)(12)^2`

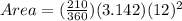
Simplifying, we get;

`Area =((210)/(360)) (3.142)(144)`

`Area =263.9 \ in^2`

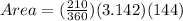
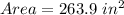
Thus, the area of the shaded sector is 263.9 square inches.

Hence, Option c is the correct answer.