Question

Lucille has a collection of more than 500 songs on her phone that have a mean duration of 215 seconds and a standard deviation of 35 seconds. Suppose that every week she makes a playlist by taking an SRS of 49 of these songs, and we calculate the sample mean duration ë of the songs in each sample. Calculate the mean and standard deviation of the sampling distribution of ________. seconds L = seconds

Answer 1

The sample mean would be:

`\mu_(\bar X) = 215 seconds`

And the deviation:

`\sigma_(\bar X) = (35)/(√(49))= 5 seconds`

Explanation:

Previous concepts

The central limit theorem states that "if we have a population with mean μ and standard deviation σ and take sufficiently large random samples from the population with replacement, then the distribution of the sample means will be approximately normally distributed. This will hold true regardless of whether the source population is normal or skewed, provided the sample size is sufficiently large".

Normal distribution, is a "probability distribution that is symmetric about the mean, showing that data near the mean are more frequent in occurrence than data far from the mean".

Solution to the problem

We know the following info for the random variable X who represent the duration

`\mu = 215, \sigma=35`

For this case we select a sampel size of n =49>30. So we can apply the central limit theorem. From the central limit theorem we know that the distribution for the sample mean
`\bar X` is given by:

`\bar X \sim N(\mu, (\sigma)/(√(n)))`

The sample mean would be:

`\mu_(\bar X) = 215 seconds`

And the deviation:

`\sigma_(\bar X) = (35)/(√(49))= 5 seconds`

Answer 2

The mean of the sampling distribution is of 215 seconds and the standard deviation is 5.

Explanation:

The Central Limit Theorem estabilishes that, for a normally distributed random variable X, with mean
`\mu` and standard deviation
`\sigma`, the sampling distribution of the sample means with size n can be approximated to a normal distribution with mean
`\mu` and standard deviation
`s = (\sigma)/(√(n))`.

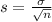For a skewed variable, the Central Limit Theorem can also be applied, as long as n is at least 30.

All songs

Mean 215 seconds, standard deviation 35 seconds

Sample

49

Mean 215, standard deviation
`s = (35)/(√(49)) = 5`

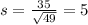
The mean of the sampling distribution is of 215 seconds and the standard deviation is 5.