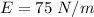Question

A +15 nC point charge is placed on the x axis at x = 1.5 m, and a -20 nC charge is placed on the y axis at y = -2.0m. What is the magnitude of the electric field at the origin?

Answer 1

Answer:
`E=75\ N/m`

Step-by-step explanation:

Given

First charge of
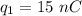`q_1=15\ nC` is placed at
`x=1.5\ m`

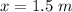
Second charge
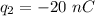`q_2=-20\ nC` is placed at
`y=-2\ m`

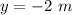
Electric field is given by

`E=(kq)/(r^2)`

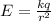
Electric field due to
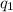`q_1` is away from it

`E_1=(9* 10^9* 15* 10^(-9))/((1.5)^2)`

`E_1=60\ N/m`

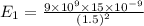
Electric field due to
`q_2`

`E_2=(9* 10^9* 20* 10^(-9))/(2^2)`

`E_2=45\ N/m`

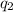
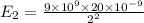
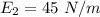
Net electric field will be vector addition of two

`\vec{E_(net)}=\vec{E_1}+\vec{E_2}`

`\vec{E_(net)}=-60\hat{i}-45\hat{j}`

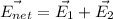
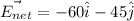
Magnitude of Electric field is

`E=√(60^2+45^2)`

`E=75\ N/m`