2 Answers

Steve Eisner · Answer 1 · 2021-01-24T11:34:01+0000

Answer:

The length of the arc is 2.25 units.

Explanation:

The circumference of a circle is
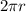$2\pi r$ , where r is radius of the circle.

Total angle of a circle in degree is 360°.

Length of central angle formula

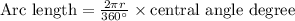
$\textrm{Arc length}=(2\pi r)/(360^\circ)* \textrm{central angle degree}$

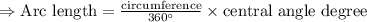
$\Rightarrow \textrm{Arc length}=\frac{\textrm{circumference}}{360^\circ}* \textrm{central angle degree}$

Given that,

A circle has a circumference of 5 units.An arc in the circle has a central angle of 162°.

Then,

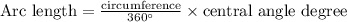
$\textrm{Arc length}=\frac{\textrm{circumference}}{360^\circ}* \textrm{central angle degree}$

$\textrm{Arc length}=(5)/(360^\circ)*162^\circ$

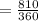
=(810)/(360)

=(9)/(4)

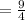
=2.25 units.

The length of the arc is 2.25 units.

Please log in or register to add a comment.