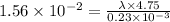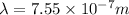Question

A laser beam is incident on two slits with a separation of 0.230 mm, and a screen is placed 4.75 m from the slits. If the bright interference fringes on the screen are separated by 1.56 cm, what is the wavelength of the laser light

Answer 1

`7.55* 10^(-7) m`

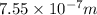
Step-by-step explanation:

We are given that

d=0.23 mm=
`0.23* 10^(-3) m`

`1mm=10^(-3) m`

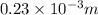
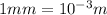
Screen is placed from the slits at distance ,L=4.75 m

The bright interference fringes on the screen are separated by 1.56 cm.

`\Delta y=1.56 cm=1.56* 10^(-2) m`

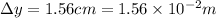
1 m=100 cm

We have to find the wavelength of laser light.

We know that

`\Delta y=(\lambda L)/(d)`

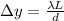
Substitute the values

`1.56* 10^(-2)=(\lambda* 4.75)/(0.23* 10^(-3))`

`\lambda=(1.56* 10^(-2)* 0.23* 10^(-3))/(4.75)`

`\lambda=7.55* 10^(-7) m`