Answer:
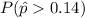
And using the z score given by:
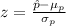
Where:


If we find the z score for
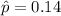 we got:
we got:
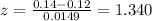
So we want to find this probability:
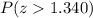
And using the complement rule and the normal standard distribution and excel we got:

Explanation:
For this case we have the proportion of interest given
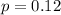 . And we have a sample size selected n = 474
. And we have a sample size selected n = 474
The distribution of
 is given by:
is given by:
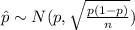
We want to find this probability:
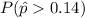
And using the z score given by:
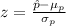
Where:


If we find the z score for
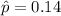 we got:
we got:
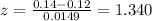
So we want to find this probability:
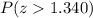
And using the complement rule and the normal standard distribution and excel we got:
