Answer:
x = 8
Explanation:
Given equation:

Start out by simplifying the distributive property on the left hand side.

Combine like terms on the left hand side.
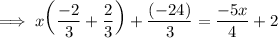
Simplify the left hand side.
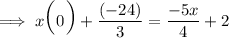
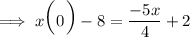
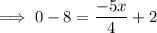
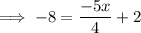
Subtract 2 both sides.
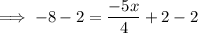
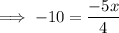
Use cross multiplication.
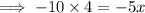
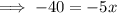
Divide -5 both sides.
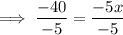
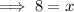
Thus, the value of x is 8.