Answer:
The river is 6 feet at two times, 2.15 hours after the rain and 4.65 hours after the rain.
Explanation:
We are given the following in the question:
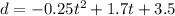
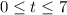
where, d is the depth of river in feet and t is time in hours after a heavy rain.
We have to find the number of hours for which the depth of river is 6 feet.
Putting d = 6 in the equation, we get,

Thus, the river is 6 feet at two times, 2.115 hours after the rain and 4.65 hours after the rain.
The attached image shows the graph.