Question

A strip of copper 130 µm thick and 4.40 mm wide is placed in a uniform magnetic field of magnitude B = 0.79 T, that is perpendicular to the strip. A current i = 26 A is then sent through the strip such that a Hall potential difference V appears across the width. Calculate V. (The number of charge carriers per unit volume for copper is 8.47 × 1028 electrons/m3.)

Answer 1

V = 1.1658 ×
`10^(-5)` V

Step-by-step explanation:

given data

strip of copper thick = 130 µm

strip of copper wide = 4.40 mm

uniform magnetic field of magnitude B = 0.79 T

current i = 26 A

number of charge carriers per unit volume = 8.47 ×
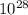`10^(28)` electrons/m³

solution

we know that number density is express as

n = \frac{Bi}{Vle} ...............1

B is uniform magnetic field and i is current and V is hall potential difference and l is thickness and e is electron charge 1.6 ×
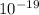`10^(-19)` C

so V will be as

V = \frac{iB}{nle} .....................2

so put here value and we get V

V =
`(26 * 0.79)/(8.47* 10^(28)* 130*10^(-6)*1.6 *10^(-19))`

V = 1.1658 ×
`10^(-5)` V