Here is the complete part of the question
A block is released from rest at height d= 40 cm and slides down a frictionless ramp and onto a first plateau, which has length d and where the coefficient of kinetic friction is 0.50. If the block is still moving, it then slides down a second frictionless ramp through height d/2 and onto a lower plateau, which has length d/2 and where the coefficient of kinetic friction is again 0.50. If the block is still moving, it then slides up a frictionless ramp until it (momentarily) stops. Where does the block stop?
Answer:
0.3 m
Step-by-step explanation:
Given that :
the height h = 40 cm = 0.40 m
Coefficient of kinetic friction is
 =0.50
=0.50
Using the Law of conservation of energy =
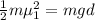
As the blocks slides down a frictionless ramp and onto a first rough plateau region.So kinetic energy is decreased to :
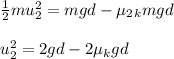
If the block is still moving, it then slides down a second frictionless ramp through an height h = d/2
Then , we can say that the gained kinetic energy is :
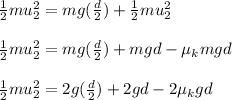
Futhermore , it moves on the horizontal surface where the coefficient of friction causes some of the kinetic energy to disappear
So, the final value of kinetic energy at the end just before climbing is :
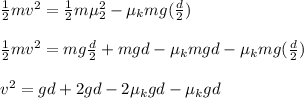
![= 3gd - 3 \mu_k gd \\ \\ = 3[g- \mu_kg ]d](https://img.qammunity.org/2021/formulas/physics/high-school/lwwp7yx7n9lv5lbpn0nfciwyn8xbnnq2ro.png)
Let represent H to be the height above the lower plateau when it momentarily stops; From the law of conservation of energy :
![(1)/(2)mv^2 = mgH \\ \\ (3)/(2)[g-\mu_kg]d = gH \\ \\ H = (3)/(2)[1-\mu_k]d \\ \\ = (3)/(2)[1-(0.50)](0.40 \ m) \\ \\ =0.3 m](https://img.qammunity.org/2021/formulas/physics/high-school/9pk4cbdg44543bavkb23nd50bhr7j4qwyd.png)