Question

A test of
`H_(0)`: μ = 20 versus
`H_(1)`: μ > 20 is performed using a significance level of ∝ = 0.05. The value of the test statistic is z = 1.47.

If the true value of μ is 25, does the test conclusion result in a Type I error, a Type II error, or a Correct decision?

Answer 1

Type II error

Explanation:

Type 1 error occurs when:

We reject a True Null Hypothesis

Type 2 error occurs when:

We fail to reject a wrong Null Hypothesis.

The given hypothesis are:

`H_(o): \mu=20\\\\ H_(a):\mu>20`

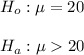
Level of significance = α = 0.05

The calculated z test statistic = z = 1.47

In order to make a decision we first need to convert z = 1.47 to its equivalent p-value. From the z-table the p value for z score being greater than 1.47 comes out to be:

p-value = 0.0708

Since, p-value is greater than the level of significance, we fail to reject the Null Hypothesis.

It is given that the true value of μ is 25. If the true value of μ is 25, then the Null hypothesis was false. But from the test we performed, we failed to reject the Null Hypothesis.

Since, we failed to reject a False Null Hypothesis, the conclusion resulted in a Type II error.