Answer:
1. 1.685
2. 16.828
Explanation:
1. log_5 15
To evaluate this logarithm you use the following formula:
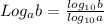
by replacing you obtain:
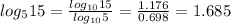
hence, the answer is 1.685
2. -3ln(1/e4/5)
to solve this logarithm you use the following properties:
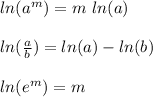
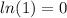
By applying all these properties you obtain:
![-3ln((1)/((e^4)/(5)))=-3[ln((1)/(e^4))-ln(5)]=-3[ln(1)-ln(e^4)-ln(5)]\\\\-3ln((1)/((e^4)/(5)))=-3[0-4-1.609]=16.828](https://img.qammunity.org/2021/formulas/mathematics/middle-school/y518jg9hu6xf04d5m9n29ksuzzfkfj2kt4.png)
hence, the answer is 16.282