Question

A roller coaster of mass 2000kg is rolling down a track with an instantaneous speed of 10m/s

Answer 1

The given is:

The given is:Mass – 2000 kg

The given is:Mass – 2000 kgInstantaneous speed – 10 meter per second

Step: 1

Step: 1K.E= 1/2mv^2

Where, K.E – Kinetic energy in joules:

m – Mass in kg

v – Velocity or speed in meter per second

Step: 2

From the give,

m = 2000 kg

v = 10 m/s

Equation (1) becomes,

K.E= 1/2 (2000) (10)^2

= (0.5)(2000)(100)

= 100000

K.E = 100,000 Joules

The kinetic energy is 100,000 Joules, if a roller coaster of mass 2000 kg is rolling down a track with an instantaneous speed 10 m/s.

Answer 2

The work done by friction on the roller coaster, with a constant force of 500 N over a distance of 30 meters, is -15,000 Joules, indicating energy loss due to opposing motion.

As the roller coaster descends the track, encountering a constant frictional force of 500 N opposing its motion, the work done by friction can be calculated using the formula:
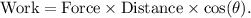`\(\text{Work} = \text{Force} * \text{Distance} * \cos(\theta)\).`Given that the distance is 30 meters and the force acts in the opposite direction of motion, the angle
`(\(\theta\))` is 180 degrees, and
`\(\cos(180^\circ) = -1\).`

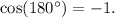
Substituting these values, we get
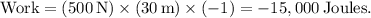`\(\text{Work} = (500 \, \text{N}) * (30 \, \text{m}) * (-1) = -15,000 \, \text{Joules}\).`The negative sign indicates that the work done by friction is in the opposite direction to the displacement, representing energy loss.

This negative work implies that the system, in this case, the roller coaster, loses energy to overcome the frictional resistance. The work-energy principle states that the work done on an object is equal to the change in its kinetic energy. In this scenario, the negative work done by friction results in a decrease in the kinetic energy of the roller coaster, illustrating the conversion of mechanical energy into non-mechanical forms due to friction. Understanding such energy transformations is crucial for analyzing the dynamics and efficiency of mechanical systems like roller coasters.