Question

vA 61.2-kg circus performer is fired from a cannon that is elevated at an angle of 57.8 ° above the horizontal. The cannon uses strong elastic bands to propel the performer, much in the same way that a slingshot fires a stone. Setting up for this stunt involves stretching the bands by 2.76 m from their unstrained length. At the point where the performer flies free of the bands, his height above the floor is the same as that of the net into which he is shot. He takes 2.60 s to travel the horizontal distance of 20.8 m between this point and the net. Ignore friction and air resistance and determine the effective spring constant of the firing mechanism.

Answer 1

The effective spring constant of the firing mechanism is 1808N/m.

Step-by-step explanation:

First, we can use kinematics to obtain the initial velocity of the performer. Since we know the angle at which he was launched, the horizontal distance and the time in which it's traveled, we can calculate the speed by:

`v_0_x=(x)/(t)\\ \\v_0\cos\theta=(x)/(t)\\\\v_0=(x)/(t\cos\theta)`

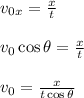
(This is correct because the horizontal motion has acceleration zero). Then:

`v_0=(20.8m)/((2.60s)\cos57.8\°)\\\\v_0=15.0m/s`

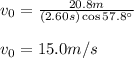
Now, we can use energy to obtain the spring constant of the firing mechanism. By the conservation of mechanical energy, considering the instant in which the elastic band is at its maximum stretch as t=0, and the instant in which the performer flies free of the bands as final time, we have:

`E_0=E_f\\\\U_e=K\\\\(1)/(2)kx^2=(1)/(2)mv^2\\\\\implies k=(mv^2)/(x^2)`

Then, plugging in the given values, we obtain:

`k=((61.2kg)(15.0m/s)^2)/((2.76m)^2)\\\\k=1808N/m`

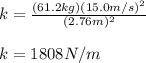
Finally, the effective spring constant of the firing mechanism is 1808N/m.