Answer:
Yes, there enough evidence to reject the claim.
Explanation:
Here we need to perform a test for single mean to determine whether the mean cost of raising a child from birth to age 2 by husband-wife families in the U.S. is $13,960.
The hypothesis is:
H₀: The mean cost of raising a child from birth to age 2 by husband-wife families in the U.S. is $13,960, i.e. µ = $13,960.
Hₐ: The mean cost of raising a child from birth to age 2 by husband-wife families in the U.S. is different from $13,960, i.e. µ ≠ $13,960.
The information provided is:
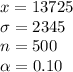
The test statistic is:

The test statistic value is -2.24.
Compute the p-value for this test statistic value at 10% level of significance as follows:
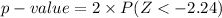

Use the z-table.
The calculated p-value is, 0.0251 < 0.10, therefore the null hypothesis will be rejected.
Conclusion:
As the null hypothesis is rejected at 10% level of significance it can be concluded that there enough evidence to reject the claim.
The mean cost of raising a child from birth to age 2 by husband-wife families in the U.S. is different from $13,960.