Question

A bat locates insects by emitting 25.3 kHz ultrasonic chirps and then listening for echoes from insects flying around it. Humans can hear sounds with frequencies up to 20 kHz. How fast would a bat have to fly and in which direction (positive away from and negative towards a stationary human listener) in order for a person to hear these chirps

Answer 1

Speed at which the bat have to fly is 90.895 m/s away from the human (listener) in positive direction.

Step-by-step explanation:

Given:

Frequency of the bat that is source here,
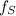`f_S` = 25.3 kHz = 25.3 * 10^3 Hz

Frequency of the listener (human),
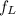`f_L` = 20 kHz = 20*10^3 Hz

We have to identify how fast the bat have to fly in order for a person to hear these chirps .

Let the velocity of bat that is source is "Vs" and "Vs" = "Vbat".

Doppler effects formulae :

• When the source is receding (moving away)
  `f_L=((V+V_L)/(V+V_S)) f_S`
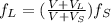
• When the source is approaching
  `f_L=((V+V_L)/(V-V_S)) f_S`
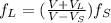
• Speed of sound in
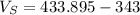  `V_S =433.895-343`the air (medium),
  `V = 343\ ms^-^1`

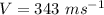
Using the above formula and considering that the bat is moving away so that the human can listen the chirps also
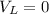`V_L=0` as listener is stationary.

⇒
`f_L=((V+V_L)/(V+V_S)) f_S` ⇒
`f_L=((V+0)/(V+V_S)) f_S`

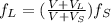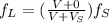
Re-arranging in terms of Vs.

⇒
`V+V_S =(V* f_S)/(f_L)`

⇒
`V_S =(V* f_S)/(f_L)-V`

⇒
`V_S =(343* 25.3* 10^3)/(20* 10^3)-343`

⇒
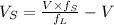
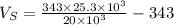
`V_S=90.895` m/s

The speed at which the bat have to fly is 90.895 m/s away from the human (listener) in positive direction.