Question

From the edge of a cliff, a 0.55 kg projectile is launched with an initial kinetic energy of 1550 J. The projectile's maximum upward displacement from the launch point is +140 m. What are the (a) horizontal and (b) vertical components of its launch velocity?

Answer 1

(a) 38.5m/s

(b) 64.4m/s

Step-by-step explanation:

First, we can obtain the launch speed from the definition of kinetic energy:

`K=(1)/(2)mv^2\\\\v=\sqrt{(2K)/(m)}\\\\`

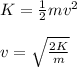
Plugging in the given values, we obtain:

`v=\sqrt{(2(1550J))/(0.55kg)}\\\\v=75.0m/s`

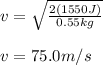
Now, from the conservation of mechanical energy, considering the instant of launch and the instant of maximum height, we get:

`E_0=E_f\\\\K_0=U_g_f+K_f\\\\(1)/(2)mv_0^2=mgh_f+(1)/(2)mv_0_x^2\\\\(1)/(2)mv_0^2=mgh_f+(1)/(2)mv_0^2\cos^2\theta\\\\\implies \cos\theta=\sqrt{1-(2gh_f)/(v_0^2)}`

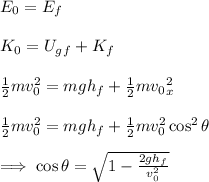
And with the known values, we compute:

`\cos\theta=\sqrt{1-(2(9.8m/s^2)(140m))/((75.0m/s)^2)}\\\\\cos\theta=0.513\\\\\theta=59.12\°`

Finally, to know the components of the launch velocity, we use trigonometry:

`v_0_x=v_0\cos\theta=(75.0m/s)\cos(59.12\°)=38.5m/s\\\\v_0_y=v_0\sin\theta=(75.0m/s)\sin(59.12\°)=64.4m/s`

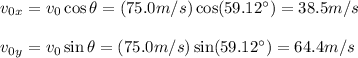
It means that the horizontal component of the launch velocity is 38.5m/s (a) and the vertical component is 64.4m/s (b).