Question

g 6. Provide an example of (a) a geometric series that diverges. (b) a geometric series PN n=0 an, that starts at n = 0 and converges. Find its sum. (c) a geometric series PN n=1 an, that starts at n = 1 and converges. Find its sum. (d) Explain how the sums for a geometric series that starts at n = 0 differs from the same series that starts at n = 1.

Answer 1

Check step-by-step-explanation.

Explanation:

A given criteria for geometric series of the form
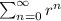`\sum_(n=0)^(\infty) r^n` is that
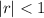`|r|<1`. Other wise, the series diverges. When it converges, we know that

`\sum_(n=0)^\infty r^n = (1)/(1-r)`.

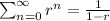So,

a)
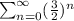`\sum_(n=0)^\infty ((3)/(2))^n` diverges since
`(3)/(2)>1`

b)
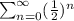`\sum_(n=0)^\infty ((1)/(2))^n`converges since
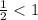`(1)/(2)<1`, and

`\sum_(n=0)^\infty ((1)/(2))^n= (1)/(1-(1)/(2)) = (2)/(2-1) = 2`

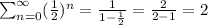
c)We can use the series in b) but starting at n=1 instead of n=0. Since they differ only on one term, we know it also converges and

`\sum_(n=1)^(\infty)((1)/(2))^n = \sum_(n=0)^(\infty)((1)/(2))^n-((1)/(2))^0 = 2-1 = 1`.

d)Based on point c, we can easily generalize that if we consider the following difference

`\sum_(n=1)^\infty r^n-\sum_(n=0)^\infty r^n = r^0 = 1`

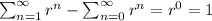
So, they differ only by 1 if the series converges.