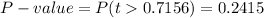Question

A sample of 20 account balances of a credit company showed an average balance of $1,170 and a standard deviation of $125. You want to determine if the mean of all account balances is significantly greater than $1,150. Assume the population of account balances is normally distributed.Compute the p-value for this test.

Answer 1

The P-value for this test is P=0.2415.

Explanation:

We have to perform an hypothesis testing on the mean of alla account balances.

The claim is that the mean of all account balances is significantly greater than $1,150.

Then, the null and alternative hypothesis are:

`H_0: \mu=1150\\\\H_a: \mu>1150`

The sample size is n=20, with a sample mean is 110 and standard deviation is 125.

We can calculate the t-statistic as:

`t=(\bar x-\mu)/(s/√(n))=(1170-1150)/(125/√(20))=(20)/(27.95)=0.7156`

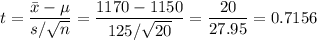
The degrees of freedom fot this test are:

`df=n-1=20-1=19`

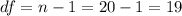
For this one-tailed test and 19 degrees of freedom, the P-value is:

`P-value=P(t>0.7156)=0.2415`