Question

A bottling operation has a mean fill level of 10.01 ounces with a standard deviation of 0.25 ounces. Random samples of 20 bottles are periodically taken to monitor the process average and the process mean is tracked using a control chart. Determine the upper and lower control limits for the chart that will include roughly 92% of the sample means when the process is in control.

Answer 1

The upper limit is 10.1

The lower limit is 9.91

Step-by-step explanation:

Given that:

The mean fill level (μ) = 10.01 ounces,

Standard deviation (σ) = 0.25 ounces

Number of sample bottles (n) = 20

The limits of the sample mean = 92% = 0.92

α = 1 - 0.92 = 0.08

`(\alpha)/(2)=0.04`

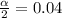
The z value of 0.04 is the same as the z value of 0.46 (0.5 - 0.04). From the probability distribution table:

`z_{(\alpha)/(2)}=z_(0.04) = 1.75`

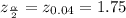
The margin of error (e) is given by:

`e=z_(0.04)(\sigma)/(√(n) )=1.75*(0.25)/(√(20) ) =0.1`

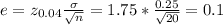
The upper limit = μ + e = 10.01 + 0.1= 10.1

The lower limit = 10.01 - 0.1 = 9.91