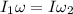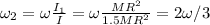Answer:
2ω/3
Step-by-step explanation:
The moments of inertia of the 1st disk is:
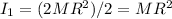
The moments of inertia of the 2nd disk is:
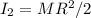
So the total moments of inertia of the system of 2 disks after the drop is:
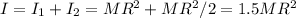
Using law of angular momentum conservation we have the following equation for before and after the drop