Question

A survey of 25 young professionals fond that they spend an average of $28 when dining out, with a standard deviation of $10. (a) Find and interpret the 95% confidence interval for the average spending. (b) Based on your answer in part (a), can you conclude statistically that the population mean is less than $30? Explain

Answer 1

a) The 95% of confidence intervals for the average spending

(23.872 , 32.128)

b) The calculated value t= 1< 1.711( single tailed test) at 0.05 level of significance with 24 degrees of freedom.

The null hypothesis is accepted

A survey of 25 young professionals statistically that the population mean is less than $30

Explanation:

Step:-(i)

Given data a survey of 25 young professionals fond that they spend an average of $28 when dining out, with a standard deviation of $10

The sample size 'n' = 25

The mean of the sample x⁻ = $28

The standard deviation of the sample (S) = $10.

Level of significance ∝=0.05

The degrees of freedom γ =n-1 =25-1=24

tabulated value t₀.₀₅ = 2.064

Step 2:-

The 95% of confidence intervals for the average spending

(
`(x^(-) - t_(\alpha ) (S)/(√(n) ) ,x^(-) + t_(\alpha )(S)/(√(n) ) )`

`(28 - 2.064 (10)/(√(25) ) ,28 + 2.064(10)/(√(25) ) )`

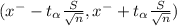
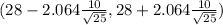
( 28 - 4.128 , 28 + 4.128)

(23.872 , 32.128)

a) The 95% of confidence intervals for the average spending

(23.872 , 32.128)

b)

Null hypothesis: H₀:μ<30

Alternative Hypothesis: H₁: μ>30

level of significance ∝ = 0.05

The test statistic

`t = (x^(-)-mean )/((S)/(√(n) ) )`

`t = (28-30 )/((10)/(√(25) ) )`

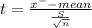
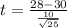
t = |-1|

The calculated value t= 1< 1.711( single tailed test) at 0.05 level of significance with 24 degrees of freedom.

The null hypothesis is accepted

Conclusion:-

The null hypothesis is accepted

A survey of 25 young professionals statistically that the population mean is less than $30