Question

Engineers want to design seats in commercial aircraft so that they are wide enough to fit 99​% of all males.​ (Accommodating 100% of males would require very wide seats that would be much too​ expensive.) Men have hip breadths that are normally distributed with a mean of 14.6 in. and a standard deviation of 1.1 in. Find Upper P 99. That​ is, find the hip breadth for men that separates the smallest 99​% from the largest 1​% by using statcrunch

Answer 1

The hip breadth for men that separates the smallest 99​% from the largest 1​% is 17.16 inches.

Explanation:

We are given that the Men have hip breadths that are normally distributed with a mean of 14.6 in. and a standard deviation of 1.1 in.

We have to find the hip breadth for men that separates the smallest 99​% from the largest 1​%.

Let X = length of hip breadths

SO, X ~ Normal(
`\mu=14.6,\sigma^(2) =1.1^(2)`)

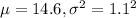The z-score probability distribution for normal distribution is given by;

Z =
`(X-\mu)/(\sigma)` ~ N(0,1)

where,
`\mu` = mean hip breadth = 14.6 inches

`\sigma` = standard deviation = 1.1 inches

The Z-score measures how many standard deviations the measure is away from the mean. After finding the Z-score, we look at the z-score table and find the p-value (area) associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X.

Now, we have to find the hip breadth for men that separates the smallest 99​% from the largest 1​%, which means;

P(X > x) = 0.01 {where x is the required hip breadth}

P(
`(X-\mu)/(\sigma)` >
`(x-14.6)/(1.1)` ) = 0.01

P(Z >
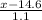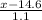`(x-14.6)/(1.1)` ) = 0.01

So, the critical value of x in the z table which represents the largest 1% of the area is given as 2.3263, that is;

`(x-14.6)/(1.1) =2.3263`

`{x-14.6}{} =2.3263* 1.1`

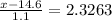
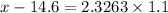
`x` = 14.6 + 2.55893 = 17.16 inches

Hence, the hip breadth for men that separates the smallest 99​% from the largest 1​% is 17.16 inches.