Question

Assume that you have paired values consisting of heights​ (in inches) and weights​ (in lb) from 40 randomly selected men. The linear correlation coefficient r is 0.5130.513. Find the value of the coefficient of determination. What practical information does the coefficient of determination​ provide?

Answer 1

The value of the coefficient of determination is 0.263 or 26.3%.

Explanation:

R-squared is a statistical quantity that measures, just how near the values are to the fitted regression line. It is also known as the coefficient of determination.

A high R² value or an R² value approaching 1.0 would indicate a high degree of explanatory power.

The R-squared value is usually taken as “the percentage of dissimilarity in one variable explained by the other variable,” or “the percentage of dissimilarity shared between the two variables.”

The R² value is the square of the correlation coefficient.

The correlation coefficient between heights​ (in inches) and weights​ (in lb) of 40 randomly selected men is:

r = 0.513.

Compute the value of the coefficient of determination as follows:

`R^(2)=(r)^(2)\\=(0.513)^(2)\\=0.263169\\\approx0.263`

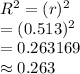
Thus, the value of the coefficient of determination is 0.263 or 26.3%.

This implies that the percentage of variation in the variable height explained by the variable weight is 26.3%.