Answer:
(a) P(

 76) = 0.2327
76) = 0.2327
(b) P(73 <
 < 75) = 0.5035
< 75) = 0.5035
(c) P(
 < 74.8) = 0.77035
< 74.8) = 0.77035
Explanation:
We are given that the mean of a population is 74 and the standard deviation is 16.
Assuming the data follows normal distribution.
Let
 = sample mean
= sample mean
The z-score probability distribution for sample mean is given by;
Z =
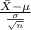 ~ N(0,1)
~ N(0,1)
where,
 = population mean = 74
= population mean = 74
 = standard deviation = 16
= standard deviation = 16
n = sample size
The Z-score measures how many standard deviations the measure is away from the mean. After finding the Z-score, we look at the z-score table and find the p-value (area) associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X.
(a) Probability that a random sample of size 34 yielding a sample mean of 76 or more is given by = P(

 76)
76)
P(

 76) = P(
76) = P(
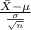

 ) = P(Z
) = P(Z
 0.73) = 1 - P(Z < 0.73)
0.73) = 1 - P(Z < 0.73)
= 1 - 0.7673 = 0.2327
The above probability is calculated by looking at the value of x = 0.73 in the z table which has an area of 0.7673.
(b) Probability that a random sample of size 120 yielding a sample mean of between 73 and 75 is given by = P(73 <
 < 75) = P(
< 75) = P(
 < 75) - P(
< 75) - P(

 73)
73)
P(
 < 75) = P(
< 75) = P(
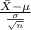 <
<
 ) = P(Z < 0.68) = 0.75175
) = P(Z < 0.68) = 0.75175
P(

 73) = P(
73) = P(
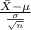

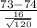 ) = P(Z
) = P(Z
 -0.68) =1 - P(Z < 0.68)
-0.68) =1 - P(Z < 0.68)
= 1 - 0.75175 = 0.24825
Therefore, P(73 <
 < 75) = 0.75175 - 0.24825 = 0.5035
< 75) = 0.75175 - 0.24825 = 0.5035
The above probability is calculated by looking at the value of x = 0.68 in the z table which has an area of 0.75175.
(c) Probability that a random sample of size 218 yielding a sample mean of less than 74.8 is given by = P(
 < 74.8)
< 74.8)
P(
 < 74.8) = P(
< 74.8) = P(
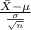 <
<
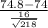 ) = P(Z < 0.74) = 0.77035
) = P(Z < 0.74) = 0.77035
The above probability is calculated by looking at the value of x = 0.74 in the z table which has an area of 0.77035.