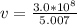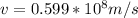Answer:
a
The the speed of light in the gemstone is
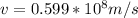
b
The unreasonable thing about this is that the speed of ligth in the gemstone is too low
the speed is 19% of the speed of light which is very low
c
One main unreasonable or inconsistent factor is that the assumption that the differnce between the angle of incidence and angle of refraction is very large
Step-by-step explanation:
From the question we are told that
The angle of incidence

The angle of refraction
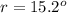
From Snell's law we have ,
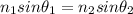
Where
 is the refractive index of the first medium (water) with a constant value of
is the refractive index of the first medium (water) with a constant value of
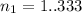
 is the refractive index of the second medium (gem stone)
is the refractive index of the second medium (gem stone)
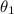 is the angle between the beam and perpendicular surface of the first medium
is the angle between the beam and perpendicular surface of the first medium
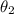 is the angle between the beam an the perpendicular surface of the second medium
is the angle between the beam an the perpendicular surface of the second medium
Making the
 the subject of the formula
the subject of the formula

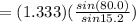
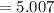
Generally refractive index of a material is mathematically represented
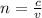
Where c is the speed light
v is the speed of light observed in a medium
Making v the subject
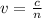
substituting value for gem stone