Answer:
The correct answer is 36.
Explanation:
An ice cream stand has 9 different flavors and 4 employees.
A group of, say x, children come to buy ice cream.
Each child buys a double scoop cone with 2 flavors and all buys different combinations.
We intend to find the number of students. But number of students is same as the number of different combinations of flavors of ice cream.
Therefore, number of possible combinations of nine ice creams with two scoops each is
![\left[\begin{array}{ccc}9\\2\end{array}\right]](https://img.qammunity.org/2021/formulas/mathematics/high-school/wzhmiwnbwitmwl2eakqyr6qjjlx8m2bdh1.png) =
=
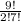 = 9 × 4 = 36
= 9 × 4 = 36
Therefore there are 36 different ways by which a child can pick up two different flavors of ice cream. Therefore, number of children, i.e. the value of x is 36.