Answer:
No, we can't reject
 at the α = 0.01 level of significance.
at the α = 0.01 level of significance.
Explanation:
We are given the sample of five matched pairs below ;
Sample 1 (B) : 20, 20, 23, 18, 22
Sample 2 (A) : 23, 16, 15, 14, 18
Let
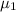 = population mean for first sample
= population mean for first sample
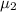 = population mean for second sample
= population mean for second sample
SO, Null Hypothesis,
 :
:
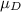 = 0 {means that there is no difference between the population means of both samples}
= 0 {means that there is no difference between the population means of both samples}
Alternate Hypothesis,
 :
:
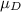 > 0 {means that three is positive difference between the population means of both samples, i.e. population mean of first sample is higher than the population mean of second sample}
> 0 {means that three is positive difference between the population means of both samples, i.e. population mean of first sample is higher than the population mean of second sample}
The test statistics that will be used here is Paired data test statistics;
T.S. =
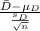 ~
~

where,
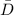 =
=
 = 20.6 - 17.2 = 3.4
= 20.6 - 17.2 = 3.4
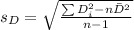 =
=
 = 3.975
= 3.975
n = sample size = 5
So, test statistics =
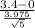 ~
~
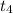
= 1.913
Now at 0.01 significance level, the t table gives critical value of 3.747 at 4 degree of freedom for right-tailed test. Since our test statistics is less than the critical value of t as 3.747 > 1.913, so we have insufficient evidence to reject our null hypothesis as it will not fall in the rejection region due to which we fail to reject our null hypothesis.
Therefore, we conclude that the there is no difference between the population means of both samples.