Question

A certain pen has been designed so that true average writing lifetime under controlled conditions (involving the use of a writing machine) is at least 10 hr. A random sample of 18 pens is selected, the writing lifetime of each is determined, and a normal probability plot of the resulting data support the use of a one-sample t test. The relevant hypotheses are H0: µ = 10 versus Ha: µ < 10.(a) If t = -2.4 and = .05 is selected, what conclusion is appropriate?a. Rejectb. Fail to reject(b) If t = -1.83 and = .01 is selected, what conclusion is appropriate?a. Rejectb. Fail to reject(c) If t = 0.57, what conclusion is appropriate?a.Rejectb. Fail to reject

Answer 1

(a) We reject our null hypothesis.

(b) We fail to reject our null hypothesis.

(c) We fail to reject our null hypothesis.

Explanation:

We are given that a certain pen has been designed so that true average writing lifetime under controlled conditions (involving the use of a writing machine) is at least 10 hr.

A random sample of 18 pens is selected.

Let
`\mu` = true average writing lifetime under controlled conditions

So, Null Hypothesis,
`H_0` :
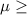`\mu \geq` 10 hr {means that the true average writing lifetime under controlled conditions is at least 10 hr}

Alternate Hypothesis,
`H_A` :
`\mu` < 10 hr {means that the true average writing lifetime under controlled conditions is less than 10 hr}

The test statistics that is used here is one-sample t test statistics;

T.S. =
`(\bar X -\mu)/((s)/(√(n) ) )` ~
`t_n_-_1`

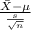where,
`\bar X` = sample mean

s = sample standard deviation

n = sample size of pens = 18

n - 1 = degree of freedom = 18 -1 = 17

Now, the decision rule based on the critical value of t is given by;

• If the value of test statistics is more than the critical value of t at 17 degree of freedom for left-tailed test, then we will not reject our null hypothesis as it will not fall in the rejection region.

• If the value of test statistics is less than the critical value of t at 17 degree of freedom for left-tailed test, then we will reject our null hypothesis as it will fall in the rejection region.

(a) Here, test statistics, t = -2.4 and level of significance is 0.05.

Now, at 0.05 significance level, the t table gives critical value of -1.74 at 17 degree of freedom.

Here, clearly the value of test statistics is less than the critical value of t as -2.4 < -1.74, so we reject our null hypothesis.

(b) Here, test statistics, t = -1.83 and level of significance is 0.01.

Now, at 0.051 significance level, the t table gives critical value of -2.567 at 17 degree of freedom.

Here, clearly the value of test statistics is more than the critical value of t as -2.567 < -1.83, so we fail to reject our null hypothesis.

(c) Here, test statistics, t = 0.57 and level of significance is not given so we assume it to be 0.05.

Now, at 0.05 significance level, the t table gives critical value of -1.74 at 17 degree of freedom.

Here, clearly the value of test statistics is more than the critical value of t as -1.74 < 0.57, so we fail to reject our null hypothesis.