Question

Integrated circuits consist of electric channels that are etched onto silicon wafers. A certain proportion of circuits are defective because of "undercutting," which occurs when too much material is etched away so that the channels, which consist of the unetched portions of the wafers, are too narrow. A redesigned process, involving lower pressure in the etching chamber, is being investigated. The goal is to reduce the rate of undercutting to less than 5%. Out of the first 1000 circuits manufactured by the new process, only 35 show evidence of undercutting. Can you conclude that the goal has been met? Find the P-value and state a conclusion.

Answer 1

There is statistical evidence to support the claim that the goal of reducing the rate of undercutting to less than 5% has been met.

P-value=0.01923.

Explanation:

We have to test the hypothesis that the proportion of defective circuits is under 5%.

Then, the null and alternative hypothesis are:

`H_0: \pi=0.05\\\\H_a:\pi<0.05`

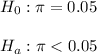
We will assume a level of significance of 0.05.

The sample, of size n=1000, has 35 defecteive circuits, so the sample proportion is:

`p=35/1000=0.035`

The standard error is calculated as if the null hypothesis is true, so it is:

`\sigma_p=\sqrt{(\pi(1-\pi))/(n)}=\sqrt{(0.05*0.95)/(1000)}}=√(0.0000475)=0.007`

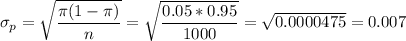
The z-statistic can be calculated as:

`z=(p-\pi+0.5/n)/(\sigma_p)=(0.035-0.050+0.5/1000)/(0.007)=(-0.0145)/(0.007)= -2.07`

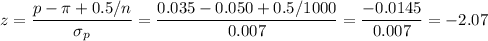
For this one-tailed test, the P-value is:

`P-value=P(z<-2.07)=0.01923`

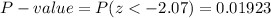
As the P-value is smaller than the significance level, the effect is significant and the null hypothesis is rejected. There is statistical evidence to support the claim that the goal of reducing the rate of undercutting to less than 5% has been met.