Question

A child, hunting for his favorite wooden horse, is running on the ground around the edge of a stationary merry-go-round. The angular speed of the child has a constant value of 0.233 rad/s. At the instant the child spots the horse, one-quarter of a turn away, the merry-go-round begins to move (in the direction the child is running) with a constant angular acceleration of 0.0136 rad/s2. What is the shortest time it takes for the child to catch up with the horse?

Answer 1

9.22 s

Step-by-step explanation:

One-quarter of a turn away is 1/4 of 2π, or π/2 which is approximately 1.57 rad

Let t (seconds) be the time it takes for the child to catch up with the horse. We would have the following equation of motion for the child and the horse:

For the child:
`s_c = \omega_ct = 0.233t`

For the horse:
`s_h = s_0 + a_ht^2/2 = 1.57 + 0.0136t^2/2 = 1.57 + 0.0068t^2`

For the child to catch up with the horse, they must cover the same angular distance within the same time t:

`s_c = s_h`

`0.233t = 1.57 + 0.0068t^2`

`0.0068t^2 - 0.233t + 1.57 = 0`

`t= (-b \pm √(b^2 - 4ac))/(2a)`

`t= (0.233\pm √((-0.233)^2 - 4*(0.0068)*(1.57)))/(2*(0.0068))`

`t= (0.233\pm0.11)/(0.0136)`

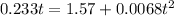
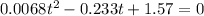
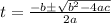
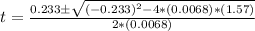
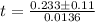
t = 25.05 or t = 9.22

Since we are looking for the shortest time we will pick t = 9.22 s