Question

When a low-pressure gas of hydrogen atoms is placed in a tube and a large voltage is applied to the end of the tube, the atoms will emit electromagnetic radiation and visible light can be observed. If this light passes through a diffraction grating, the resulting spectrum appears as a pattern of four isolated, sharp parallel lines, called spectral lines. Each spectral line corresponds to one specific wavelength that is present in the light emitted by the source. Such a discrete spectrum is referred to as a line spectrum. By the early 19th century, it was found that discrete spectra were produced by every chemical element in its gaseous state. Even though these spectra were found to share the common feature of appearing as a set of isolated lines, it was observed that each element produces its own unique pattern of lines. This indicated that the light emitted by each element contains a specific set of wavelengths that is characteristic of that element.

Answer 1

Complete Question

The complete question is shown on the first uploaded image

Answer:

The value of n is
`n =7`

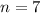
Step-by-step explanation:

From the question we are told that

The value of m = 2

For every value of
`m, n = m+ 1, m+2,m+3,....`

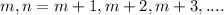
The modified version of Balmer's formula is
`(1)/(\lambda) = R [(1)/(m^2) - (1)/(n^2) ]`

The Rydberg constant has a value of
`R = 1.097 *10^(7) m^(-1)`

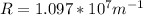
The objective of this solution is to obtain the value of n for which the wavelength of the Balmer series line is smaller than 400nm

For m = 2 and n =3

The wavelength is

`(1)/(\lambda ) = (1.097 * 10^7)[(1)/(2^2) - (1)/(3^2) ]`

`\lambda = (1)/(1523611.1112)`

`\lambda = 656nm`

For m = 2 and n = 4

The wavelength is

`(1)/(\lambda ) = (1.097 * 10^7)[(1)/(2^2) - (1)/(4^2) ]`

`\lambda = (1)/(2056875)`

`\lambda = 486nm`

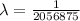
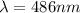
For m = 2 and n = 5

The wavelength is

`(1)/(\lambda ) = (1.097 * 10^7)[(1)/(2^2) - (1)/(5^2) ]`

`\lambda = (1)/(2303700)`

`\lambda = 434nm`

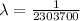
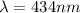
For m = 2 and n = 6

The wavelength is

`(1)/(\lambda ) = (1.097 * 10^7)[(1)/(2^2) - (1)/(6^2) ]`

`\lambda = (1)/(2422222)`

`\lambda = 410nm`

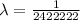
For m = 2 and n = 7

The wavelength is

`(1)/(\lambda ) = (1.097 * 10^7)[(1)/(2^2) - (1)/(7^2) ]`

`\lambda = (1)/(2518622)`

`\lambda = 397nm`

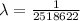
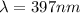
So the value of n is 7