Answer with Step-by-step explanation:
We are given that

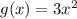
f(x) is linear function
Domain of f(x)=R
g(x) is a quadratic function
Domain of g(x)=R
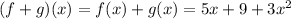
Domain of (f+g)=R
(f-g)(x)=f(x)-g(x)=
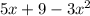
Domain of (f-g)=R
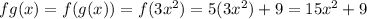
Domain of fg=R
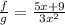
The function is not defined where g(x)=0
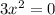

Domain of f/g=R-{0}