Answer:
(7, 19)
Explanation:
We know that we are talking about circle C, which means that the center is point C. Since the diameter is AB, that means that AB goes through the center C and that center C is the midpoint of the segment AB.
Say the coordinates of B are (x, y). We can use the Midpoint Theorem to figure out B. The Midpoint Theorem states that for two points
 and
and
 , the coordinates of the midpoint are:
, the coordinates of the midpoint are:
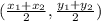 .
.
Here, our two points are A(5, 1) and B(x, y). Then, the midpoint is:
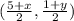 . We already know the midpoint is C(6, 10), so we can just set 6 equal to
. We already know the midpoint is C(6, 10), so we can just set 6 equal to
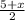 and set 10 equal to
and set 10 equal to
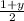 :
:
6 =
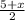 ⇒ 12 = 5 + x ⇒ x = 12 - 5 = 7
⇒ 12 = 5 + x ⇒ x = 12 - 5 = 7
AND
10 =
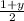 ⇒ 20 = 1 + y ⇒ y = 20 - 1 = 19
⇒ 20 = 1 + y ⇒ y = 20 - 1 = 19
So, the coordinates of B is: (7, 19).
Hope this helps!