Question

A 3.5-m3 rigid tank initially contains air whose density is 2 kg/m3 . The tank is connected to a high-pressure supply line through a valve. The valve is opened, and air is allowed to enter the tank until the density in the tank rises to 6.5 kg/m3 . Determine the mass of air that has entered the tank.

Answer 1

Step-by-step explanation:

First, we find the mass of the air originally in the tank.

Density is given as mass divided by volume. It is given as:

`Density = (mass)/(volume)`

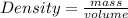
Therefore, mass is:

`mass = denisty *volume`

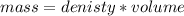
Density of air =
`2 kg/m^3`; Volume of the tank =
`3.5 m^3`

`=> Mass = 3.5 * 2 = 7 kg`

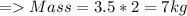
The mass of the air initially in the tank is 7 kg.

After air is allowed to enter, the mass changes.

New density =
`6.5 kg/m^3`

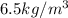
The new mass will be:

`Mass = 6.5 * 3.5 = 22.75 kg`

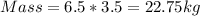
We can now find the mass of air that has entered the tank:

Mass of air that entered tank = New mass of air - Original mass of air

M = 22.75 - 7.0 = 15.75 kg

The mass of air that entered the tank is 15.75 kg.