Answer:
99.5% of all samples of 21 of today's women in their 20's have mean heights of at least 65.86 inches.
Explanation:
We are given that a half-century ago, the mean height of women in a particular country in their 20's was 64.7 inches. Assume that the heights of today's women in their 20's are approximately normally distributed with a standard deviation of 2.07 inches.
Also, a samples of 21 of today's women in their 20's have been taken.
Let
 = sample mean heights
= sample mean heights
The z-score probability distribution for sample mean is given by;
Z =
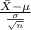 ~ N(0,1)
~ N(0,1)
where,
 = population mean height of women = 64.7 inches
= population mean height of women = 64.7 inches
 = standard deviation = 2.07 inches
= standard deviation = 2.07 inches
The Z-score measures how many standard deviations the measure is away from the mean. After finding the Z-score, we look at the z-score table and find the p-value (area) associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X.
Now, Probability that the sample of 21 of today's women in their 20's have mean heights of at least 65.86 inches is given by = P(

 65.86 inches)
65.86 inches)
P(

 65.86 inches) = P(
65.86 inches) = P(
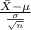

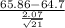 ) = P(Z
) = P(Z
 -2.57) = P(Z
-2.57) = P(Z
 2.57)
2.57)
= 0.99492 or 99.5%
The above probability is calculated by looking at the value of x = 2.57 in the z table which has an area of 0.99492.
Therefore, 99.5% of all samples of 21 of today's women in their 20's have mean heights of at least 65.86 inches.