Question

"Suppose that an automobile manufacturer advertises that its new hybrid car has a mean gas mileage of 50 miles per gallon. You take a simple random sample of 30 hybrid vehicles and test their gas mileage. You find that in this sample, the average is x = 47 miles per gallon with a standard deviation of s = 5.5 miles per gallon. Does this indicate that the advertiser’s statement is too high, with  = 0.1 ?"

Answer 1

We conclude that the new hybrid car has a mean gas mileage of less than 50 miles per gallon which means that the advertiser’s statement is too high.

Explanation:

We are given that an automobile manufacturer advertises that its new hybrid car has a mean gas mileage of 50 miles per gallon.

A simple random sample of 30 hybrid vehicles is taken and tested their gas mileage. It was found that in this sample, the average is x = 47 miles per gallon with a standard deviation of s = 5.5 miles per gallon.

Let
`\mu` = mean gas mileage of new hybrid car.

SO, Null Hypothesis,
`H_0` :
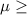`\mu \geq` 50 miles per gallon {means that the new hybrid car has a mean gas mileage of more than or equal to 50 miles per gallon}

Alternate Hypothesis,
`H_A` :
`\mu` < 50 miles per gallon {means that the new hybrid car has a mean gas mileage of less than 50 miles per gallon}

The test statistics that will be used here is One-sample t test statistics as we don't know about the population standard deviation;

T.S. =
`(\bar X -\mu)/((s)/(√(n) ) )` ~
`t_n_-_1`

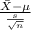where,
`\bar X` = sample average gas mileage = 47 miles per gallon

s = sample standard deviation = 5.5 miles per gallon

n = sample of hybrid vehicles = 30

So, test statistics =
`(47-50)/((5.5)/(√(30) ) )` ~
`t_2_9`

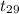
= -2.988

Now at 0.1 significance level, the t table gives critical value of -1.311 at 29 degree of freedom for left-tailed test. Since our test statistics is less than the critical value of t as -1.311 > -2.988, so we have sufficient evidence to reject our null hypothesis as it will fall in the rejection region due to which we reject our null hypothesis.

Therefore, we conclude that the new hybrid car has a mean gas mileage of less than 50 miles per gallon which means that the advertiser’s statement is too high.