Question

A company with a fleet of 150 cars found that the emissions systems of only 4 out of the 25 they tested failed to meet pollution control guidelines. The company initially believed that 30% of the fleet was out of compliance. Is this strong evidence the percentage of the fleet out of compliance is different from their initial thought

Answer 1

No, there is no strong evidence that the percentage of the fleet out of compliance is different from their initial thought.

Explanation:

We are given that a company with a fleet of 150 cars found that the emissions systems of only 4 out of the 25 they tested failed to meet pollution control guidelines.

The company initially believed that 30% of the fleet was out of compliance.

Let p = percentage of the fleet that was out of compliance.

SO, Null Hypothesis,
`H_0` : p = 30% {means that the percentage of the fleet out of compliance is same as their initial thought}

Alternate Hypothesis,
`H_A` : p
`\\eq` 30% {means that the percentage of the fleet out of compliance is different from their initial thought}

The test statistics that will be used here is One-sample z proportion statistics;

T.S. =
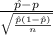`\frac{\hat p-p}{{\sqrt{(\hat p(1-\hat p))/(n) } } } }` ~ N(0,1)

where,
`\hat p` = percentage of the fleet out of compliance =
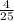`(4)/(25)` = 16%

n = sample of systems tested = 25

So, test statistics =
`\frac{0.16-0.30}{{\sqrt{(0.16(1-0.16))/(25) } } } }`

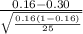
= -1.909

The value of the test statistics is -1.909.

Since in the question we are not given with the level of significance at which hypothesis can be tested, so we assume it to be 5%. Now at 5% significance level, the z table gives critical values between -1.96 and 1.96 for two-tailed test.

Since our test statistics lies within the range of critical values of z, so we have insufficient evidence to reject our null hypothesis as it will not fall in the rejection region due to which we fail to reject our null hypothesis.

Therefore, we conclude that the percentage of the fleet out of compliance is same as their initial thought.